Learn about point discontinuities, removable discontinuities, jump.
Give one value of a where the limit can be solved using direct evaluation. A removable discontinuity exists when the limit of the function exists, but one or both of the other two conditions is not met. Stack exchange network consists of 178 q&a communities including stack overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. To determine if the discontinuity at x equals negative two is removable; The function f (x) has a discontinuity of the first kind at x = a if.
The removable discontinuity can be given as:
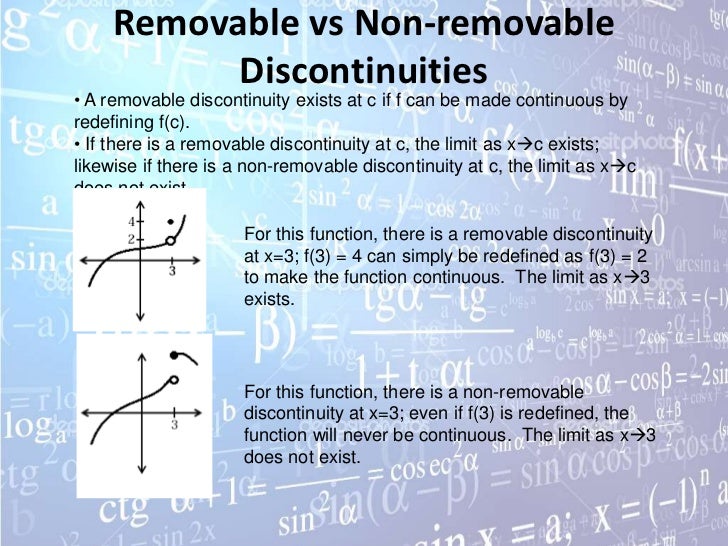
Types of discontinuities (i) removable (ii) jump (iii) infinite nonremovable at a particular point we can classify three types of discontinuities. ^removable discontinuity _ discontinuous at this point the limit of the left is not equal to the limit from the right ^jump discontinuity _ discontinuous at this point the limit from the left is equal to the right, but is not equal to the value of the function ^removable discontinuity _ continuous at this point If x is negative, though, it changes the sign on top and the top function x, divided by x, is one. A removable discontinuity exists when the limit of the function exists, but one or both of the other two conditions is not met. The limit of a removable discontinuity is simply the value the function would take at that discontinuity if it were not a discontinuity. From one piece of the So the limits one the bottom one negative x divided by x, is negative one. On the other hand, if adjusting a function's value at a specific point of discontinuity will give a continuous function, then we. We actually evaluated this limit expression using a graphical approach earlier in the unit. Suppose a function is undefined at a certain real value like {eq}x=a {/eq}; Lim xa f (x) does not exist. Otherwise, has a discontinuity at. This time the hole could be filled by redefining the point with an x coordinate of 2 so that it fills the hole.
We actually evaluated this limit expression using a graphical approach earlier in the unit. In other words, $\lim\limits_{x\to c+}f(x)=\infty$, or one of the other three varieties of infinite limits. The next step would be to check if these factors also appear in. If all three of these are equal, then is continuous at. For instance, the function f(x) = (x — 1)^2 / (x — 1) has a limit equal to 0 as x goes to 1, but is not defined at x.

Stack exchange network consists of 178 q&a communities including stack overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers.
For instance, the function f(x) = (x — 1)^2 / (x — 1) has a limit equal to 0 as x goes to 1, but is not defined at x. The graph of f has a horizontal asymptote at y 3, and f has a removable discontinuity at x 2 (a) show that a 6 and b 13 (b) to make f continuous at x 2 f 2 should be defined as what value? removable discontinuities occur when a rational function has a factor with an x that exists in both the numerator and the denominator. F (x)= x if x<= 1, f (x)= x+ 2 if x> The function is not continuous because there is a jump or because there is an infinite portion which leads to an asymptote. The value that would make the function continuous at a removable discontinuity is the limit of the function at the point. You will define continuous in a more mathematically rigorous way after you study limits. Room to graph each problem is provided. The function is not continuous because there is a hole.in this case, all limits exist. In this limits worksheet, students evaluate limits and graph given expressions. There is infinite discontinuity when one of the unilateral limits of function is infinite. Figure illustrates the differences in these types of. They occur when factors can be algebraically canceled from rational functions.
Lim xa f (x) does not exist. This time the hole could be filled by redefining the point with an x coordinate of 2 so that it fills the hole. • at x =6, the function has an infinite discontinuity. The limit must exist and be finite, or else we would have a jump or asymptotic discontinuity. An essential discontinuity can't be quantified.
There is infinite discontinuity when one of the unilateral limits of function is infinite.
Functions that can be drawn without lifting up your pencil are called continuous functions. A jump discontinuity at a point has limits that exist, but it's different on both sides of the gap. Formally, a removable discontinuity is one at which the limit of the function exists but does not equal the value of the function at that point; The function is not continuous because there is a jump or because there is an infinite portion which leads to an asymptote. Figure illustrates the differences in these types of. This time the hole could be filled by redefining the point with an x coordinate of 2 so that it fills the hole. When a function is not continuous at a point, then we can say it is discontinuous at that point. It is clear that there will be some form of a discontinuity at x=1 (as there the denominator is 0). If the limit does not exist. If there is a finite limit at a, but it is not the same as the value of the function, then the function has a removable discontinuity. The value that would make the function continuous at a removable discontinuity is the limit of the function at the point. This is an example of a removable discontinuity, since is a finite number. This worksheet is a great springboard into a conversation about limits and continuity.
Get Removable Discontinuity Limits Pictures. ^removable discontinuity _ discontinuous at this point the limit of the left is not equal to the limit from the right ^jump discontinuity _ discontinuous at this point the limit from the left is equal to the right, but is not equal to the value of the function ^removable discontinuity _ continuous at this point A discontinuity is "not removeable" Stack exchange network consists of 178 q&a communities including stack overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. The limit from the right does not exist. There are three types of discontinuities: