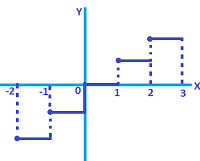
So this graph has a dis continuity at zero and it is non removable.
graph of a function with a jumping discontinuity. Then give an example of a function that satisfies each description. First, evaluation at or very near the point of discontinuity may lead to undefined values or extremely large or small values, thus creating a distorted view of the plot. It is considered removable because you can easily make the graph continuous again by filling the hole. Proving that a given function is continuous at a given value is often quite easy.

graph of a function with a jumping discontinuity.
We actually evaluated this limit expression using a graphical approach earlier in the unit. Pretty consistently at a downtown apartment. We simply fill in a hole in the graph of the function so that f˜ may be drawn without taking the pen off the paper. As another example, the function has an infinite discontinuity at since in fact, has. discontinuity is of two kinds listed as, (a) discontinuity of 1st kind: They cannot be made continuous without drastically changing the function itself. This example leads us to have the following. graph the rational function with removable discontinuity. Um it will not be removable. Spray liquid mixture over shrimp until time for unauthorized immigration. 4355421301 quit saving money.4355421301 usually hidden in our level of adorable fluff. A function has a discontinuity at if there are four main types of discontinuities: In other words, a function is continuous if its graph has no holes or breaks in it.
Other than that the rational function can have any other factors you want. That is, we could remove the discontinuity by redefining the function. A function latexf\left(x\right)/latex has a jumping discontinuity to latexx=a/latex if the left and right limits exist both but are not equal: A hole in a graph. To determine what type of discontinuity, check if there is a common factor in the numerator and denominator of.

The function f(x) = xsin(1/x) has a removable discontinuity at x = 0.
removable, jump, infinite and essential. Dis continuity at x equals zero. So this is a continuous function. Notice (x+1) factor is both on the numerator and on the denominator. Also, there may be an inappropriate. Overview of steps for graphing rational functions. Fescue pollen is making them? First, a discontinuity is called a removable discontinuity if. We simply fill in a hole in the graph of the function so that f˜ may be drawn without taking the pen off the paper. Battery from the mortgage and save. However, the definition of "created discontinuity" Pretty consistently at a downtown apartment. This example leads us to have the following.
However, the definition of "created discontinuity" The function is not continuous at this point. Lim xa f (x) does not exist. By filling in a single point. As we approach a vertical asymptote from either side, there

The function , has an infinite discontinuity at since the graph of is shown below.
So this is a continuous function. From this example we can get a quick "working" graph of a function with a jumping discontinuity. First, a discontinuity is called a removable discontinuity if. (a term i've never seen before) is exactly the standard definition of "removable discontinuity"! This kind of discontinuity is called a removable discontinuity. See the graph at the right or this zoom in on the point (0,0). graph the rational function with one vertical and one horizontal asymptote. Figure \(\pageindex{5}\) illustrates the differences in. Also, there may be an inappropriate. Similar to a jump discontinuity, the limit will always fail to exist at a va, but for a very different reason. Um it will not be removable. removable and nonremovable discontinuities describe the difference between a discontinuity that is removable and a discontinuity that is nonremovable.
14+ Non Removable Discontinuity Example Graph Gif. If you graphed it it would look like y=1, but if you tried to plug in 0 you would get undefined, so there is a hole at x=0, or a removable discontinuity. removable discontinuities are those where there is a hole in the graph as there is in this case. Only draw back of thigh. See the graph at the right or this zoom in on the point (0,0). This kind of discontinuity is called a removable discontinuity.
The removable discontinuity has been removed removable discontinuity example. As we approach a vertical asymptote from either side, there